Understanding Imaginary and Complex Numbers

Imaginary and complex numbers are fascinating mathematical concepts that extend the number system beyond real numbers. These numbers have wide-ranging applications in various fields, including engineering, physics, and computer science. In this article, we will dive into the definitions of imaginary and complex numbers, explore their properties, and discuss how they are used in both theoretical and applied mathematics.

What are Imaginary Numbers?

Imaginary numbers arise when we try to find the square root of a negative number, which is not possible within the set of real numbers. To handle such cases, mathematicians defined a new unit, denoted by the symbol $i$, where:

Using this definition, we can express the square root of any negative number. For example:

Thus, an imaginary number is a number of the form $bi$, where $b$ is a real number and $i$ represents the imaginary unit.

What are Complex Numbers?

A complex number is a combination of a real part and an imaginary part, and it is written in the form:

Where:

• $a$ is the real part,
• $bi$ is the imaginary part,
• $i$ is the imaginary unit, satisfying $i^2 = -1$.

The set of all complex numbers is denoted by $\mathbb{C}$, and it extends the real number system to include numbers of the form $a + bi$. When $b = 0$, the complex number is purely real, and when $a = 0$, the number is purely imaginary.

Examples of Complex Numbers:

• $z = 3 + 4i$ (real part = 3, imaginary part = 4).
• $z = -5i$ (purely imaginary, real part = 0).
• $z = 7$ (purely real, imaginary part = 0).

Operations with Complex Numbers

Just like real numbers, complex numbers can be added, subtracted, multiplied, and divided. Let’s explore each of these operations.

1. Addition and Subtraction

To add or subtract complex numbers, simply combine the real parts and the imaginary parts separately.

Addition: If $z_1 = a + bi$ and $z_2 = c + di$, then:

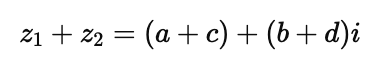
Example: Add $z_1 = 2 + 3i$ and $z_2 = 4 + 5i$.

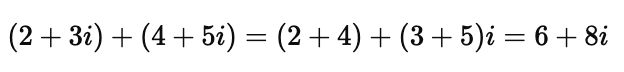
Subtraction: Similarly, for subtraction:

Example: Subtract $z_2 = 4 + 5i$ from $z_1 = 2 + 3i$.

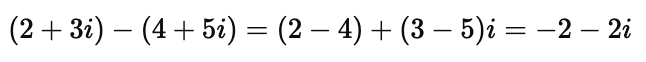
2. Multiplication

To multiply two complex numbers, apply the distributive property (also known as the FOIL method for binomials) and use the fact that $i^2 = -1$.

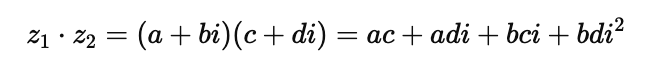
Since $i^2 = -1$, this simplifies to:

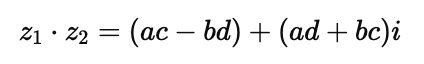
Example: Multiply $z_1 = 2 + 3i$ and $z_2 = 4 + 5i$.

3. Division

To divide two complex numbers, multiply the numerator and denominator by the complex conjugate of the denominator. The complex conjugate of $z = a + bi$ is $a – bi$, and multiplying a complex number by its conjugate gives a real number.

Example: Divide $z_1 = 2 + 3i$ by $z_2 = 4 + 5i$.

First, find the complex conjugate of $z_2 = 4 + 5i$, which is $4 – 5i$. Then multiply both the numerator and denominator by $4 – 5i$:

Simplify the numerator and denominator:

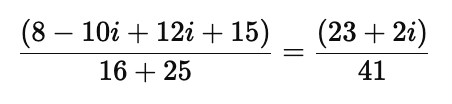
Thus, the result is:

Absolute Value (Modulus) of a Complex Number

The absolute value or modulus of a complex number $z = a + bi$ is its distance from the origin in the complex plane, and it is denoted by $|z|$. The modulus is calculated as:

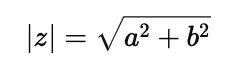
Example: Find the modulus of $z = 3 + 4i$.

Complex Conjugate

The complex conjugate of a complex number $z = a + bi$ is $a – bi$. The complex conjugate is useful in operations like division, as seen earlier, and it also has properties related to the modulus:

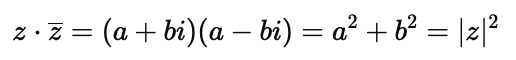
The Complex Plane

Complex numbers can be represented visually using the complex plane (also known as the Argand plane). In this plane:

• The horizontal axis represents the real part of a complex number,
• The vertical axis represents the imaginary part.

A complex number $z = a + bi$ is plotted as the point $(a, b)$ on the complex plane. The distance from the origin to the point $(a, b)$ is the modulus $|z|$, and the angle between the positive real axis and the line connecting the origin to $(a, b)$ is called the argument of the complex number.

Polar Form of Complex Numbers

Complex numbers can also be expressed in polar form, which uses the modulus $|z|$ and the argument $\theta$. The polar form of a complex number is:

Where:

• $r = |z|$ is the modulus,
• $\theta$ is the argument, or the angle the complex number makes with the positive real axis.

This is sometimes written as:

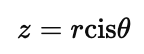
Polar form is particularly useful for multiplying and dividing complex numbers, as well as for raising them to powers.

Example of Polar Form

Let $z = 3 + 4i$. To express this in polar form:

Find the modulus $r$:

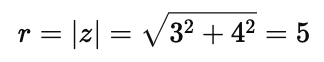
Find the argument $\theta$:

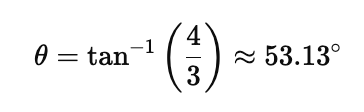
Thus, in polar form:

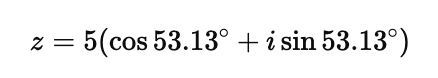
Applications of Complex Numbers

Complex numbers are used in various fields due to their ability to describe phenomena that real numbers cannot.

1. Electrical Engineering

In electrical engineering, complex numbers are used to analyze alternating current (AC) circuits. The voltage, current, and impedance in an AC circuit are often represented as complex numbers, where the real part corresponds to the resistive component, and the imaginary part corresponds to the reactive component.

2. Signal Processing

In signal processing, complex numbers are used to represent waveforms, such as sound or electromagnetic waves. The Fourier transform, a fundamental tool in signal processing, uses complex numbers to break down a signal into its frequency components.

3. Quantum Mechanics

In quantum mechanics, the state of a quantum system is described by complex-valued wave functions. These wave functions encode information about probabilities, which are found by taking the modulus of the complex numbers involved.

4. Fractals and Computer Graphics

Complex numbers play a crucial role in generating fractals, such as the famous Mandelbrot set. The iterative process used to create these intricate patterns involves complex arithmetic.

Common Mistakes to Avoid

1. Confusing $i$ with a variable: Remember that $i$ is a constant that satisfies $i^2 = -1$, not a variable.
2. Incorrect handling of complex conjugates: Always remember to change the sign of the imaginary part when calculating the conjugate.
3. Forgetting to simplify expressions: When multiplying complex numbers, don’t forget to replace $i^2$ with $-1$.

Conclusion

Imaginary and complex numbers extend the familiar number system in powerful ways, opening doors to new mathematical insights and practical applications. From solving equations that have no real solutions to analyzing electrical circuits and quantum systems, complex numbers provide a rich framework for understanding and modeling the world. By mastering the operations and properties of these numbers, you’ll be equipped to tackle a wide range of problems in both pure and applied mathematics.