The unit circle is a powerful tool in trigonometry, providing a visual and conceptual framework for understanding angles, radians, and trigonometric functions. Mastering the unit circle simplifies complex calculations and helps you grasp the relationships between sine, cosine, and tangent values. In this article, we will explore the basics of the unit circle, explain its significance, and show how it connects to trigonometric functions and identities.
What is the Unit Circle?

The unit circle is a circle with a radius of 1 unit, centered at the origin of the coordinate plane, where the coordinates of points on the circle correspond to angles and trigonometric values. Mathematically, the equation of the unit circle is:

In this equation:
- The coordinates $(x, y)$ represent any point on the circle.
- The radius of the circle is always 1.
By defining angles on this circle, we can understand how the coordinates of these points relate to the trigonometric functions sine and cosine.
Angles on the Unit Circle
Angles can be measured in two ways:
- Degrees: A unit of angular measure where a full circle is $360^\circ$.
- Radians: A unit of angular measure where a full circle is $2\pi$ radians.
The key angles to remember on the unit circle are commonly found at:
- $0^\circ$ or $0$ radians,
- $90^\circ$ or $\frac{\pi}{2}$ radians,
- $180^\circ$ or $\pi$ radians,
- $270^\circ$ or $\frac{3\pi}{2}$ radians,
- $360^\circ$ or $2\pi$ radians.
These standard positions on the unit circle correspond to key points where the trigonometric values of sine and cosine are easy to calculate.
Trigonometric Functions on the Unit Circle
On the unit circle, the coordinates of any point $(x, y)$ represent the cosine and sine of the angle $\theta$ formed with the positive $x$-axis. Specifically:
- The x-coordinate corresponds to $\cos(\theta)$,
- The y-coordinate corresponds to $\sin(\theta)$.
Thus, for any angle $\theta$:
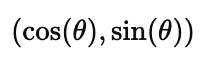
The tangent function can also be derived from the unit circle, defined as the ratio of sine to cosine:
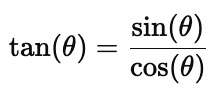
Key Trigonometric Values on the Unit Circle
For certain standard angles, the sine and cosine values are well known and frequently used in trigonometry. These angles include $0^\circ$, $30^\circ$, $45^\circ$, $60^\circ$, and $90^\circ$, or their radian equivalents.
Here’s a list of common angles and their sine and cosine values:
| Angle ($\theta$) | Radians | $\cos(\theta)$ | $\sin(\theta)$ |
|---|---|---|---|
| $0^\circ$ | $0$ | $1$ | $0$ |
| $30^\circ$ | $\frac{\pi}{6}$ | $\frac{\sqrt{3}}{2}$ | $\frac{1}{2}$ |
| $45^\circ$ | $\frac{\pi}{4}$ | $\frac{\sqrt{2}}{2}$ | $\frac{\sqrt{2}}{2}$ |
| $60^\circ$ | $\frac{\pi}{3}$ | $\frac{1}{2}$ | $\frac{\sqrt{3}}{2}$ |
| $90^\circ$ | $\frac{\pi}{2}$ | $0$ | $1$ |
Symmetry on the Unit Circle
The unit circle has symmetry that allows you to easily find trigonometric values for angles in all four quadrants:
- First Quadrant ($0^\circ$ to $90^\circ$ or $0$ to $\frac{\pi}{2}$): Both sine and cosine are positive.
- Second Quadrant ($90^\circ$ to $180^\circ$ or $\frac{\pi}{2}$ to $\pi$): Sine is positive, cosine is negative.
- Third Quadrant ($180^\circ$ to $270^\circ$ or $\pi$ to $\frac{3\pi}{2}$): Both sine and cosine are negative.
- Fourth Quadrant ($270^\circ$ to $360^\circ$ or $\frac{3\pi}{2}$ to $2\pi$): Sine is negative, cosine is positive.
This symmetry means you can easily determine the sine and cosine values of angles beyond $90^\circ$ by recognizing the relationship between the quadrants and their reference angles (the corresponding acute angle in the first quadrant).
Radians vs. Degrees: Understanding the Conversion
Radians and degrees are two different ways to measure angles, but they are directly related through the following conversion factor:

Using this, you can convert between radians and degrees:
To convert degrees to radians:
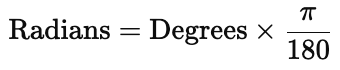
To convert radians to degrees:
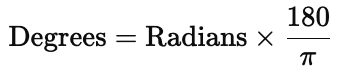
For example, to convert $60^\circ$ to radians:
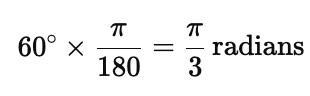
Using the Unit Circle to Solve Trigonometric Equations
The unit circle is a powerful tool for solving trigonometric equations. For example, consider the equation:

From the unit circle, you know that $\sin(\theta) = \frac{\sqrt{3}}{2}$ at $\theta = 60^\circ$ (or $\frac{\pi}{3}$ radians) in the first quadrant. However, since sine is also positive in the second quadrant, the solution also includes $\theta = 120^\circ$ (or $\frac{2\pi}{3}$ radians).
Thus, the two solutions in degrees are:

Trigonometric Identities Derived from the Unit Circle
Several important trigonometric identities can be visualized and derived using the unit circle:
- Pythagorean Identity: Since the unit circle has a radius of 1, we know that:
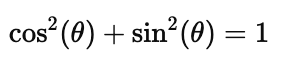
This identity holds true for any angle $\theta$.
- Reciprocal Identities:
- $\csc(\theta) = \frac{1}{\sin(\theta)}$
- $\sec(\theta) = \frac{1}{\cos(\theta)}$
- $\cot(\theta) = \frac{1}{\tan(\theta)}$
- Even-Odd Identities:
- $\sin(-\theta) = -\sin(\theta)$ (Sine is an odd function.)
- $\cos(-\theta) = \cos(\theta)$ (Cosine is an even function.)
Applications of the Unit Circle
The unit circle is not just a theoretical concept—it has a wide range of practical applications in fields like engineering, physics, and even everyday life.
1. Wave Motion and Oscillations
Trigonometric functions derived from the unit circle are used to model wave motion, such as sound waves, light waves, and electrical oscillations. The sine and cosine functions describe periodic phenomena that repeat in regular cycles, making them essential in signal processing and communication technologies.
2. Rotational Motion
In physics, the unit circle helps describe the rotational motion of objects. Angular displacement, velocity, and acceleration are all modeled using trigonometric functions, and the unit circle provides a clear visualization of these concepts.
3. Circular Motion
In mechanics, the unit circle helps describe objects in uniform circular motion. The x and y coordinates of the object’s position on the circle correspond to its cosine and sine values, respectively, making it easier to calculate velocity and acceleration in circular paths.
4. Computer Graphics
In computer graphics, the unit circle is used to model rotations, transformations, and the behavior of objects in 2D and 3D spaces. The relationship between angles and coordinates is essential for rendering realistic animations and simulations.
Common Mistakes to Avoid
- Forgetting Radians: In trigonometry, always ensure that your calculator is set to the correct mode (radians or degrees) based on the context of the problem.
- Misplacing Signs in Different Quadrants: Keep track of which quadrant you’re in. In the second and third quadrants, trigonometric functions change signs. For example, sine is positive in the second quadrant but negative in the third.
- Ignoring the Unit Circle Symmetry: Don’t forget that the unit circle’s symmetry allows you to quickly find trigonometric values for angles greater than $90^\circ$ by using reference angles.
Conclusion
The unit circle is a foundational concept in trigonometry that simplifies the understanding of angles, radians, and trigonometric functions. By mastering the unit circle, you gain a powerful tool for solving trigonometric equations, deriving identities, and understanding periodic phenomena in real-world applications. Whether you’re a student or a professional, the unit circle opens the door to a deeper comprehension of the mathematical relationships that govern motion, waves, and rotation.

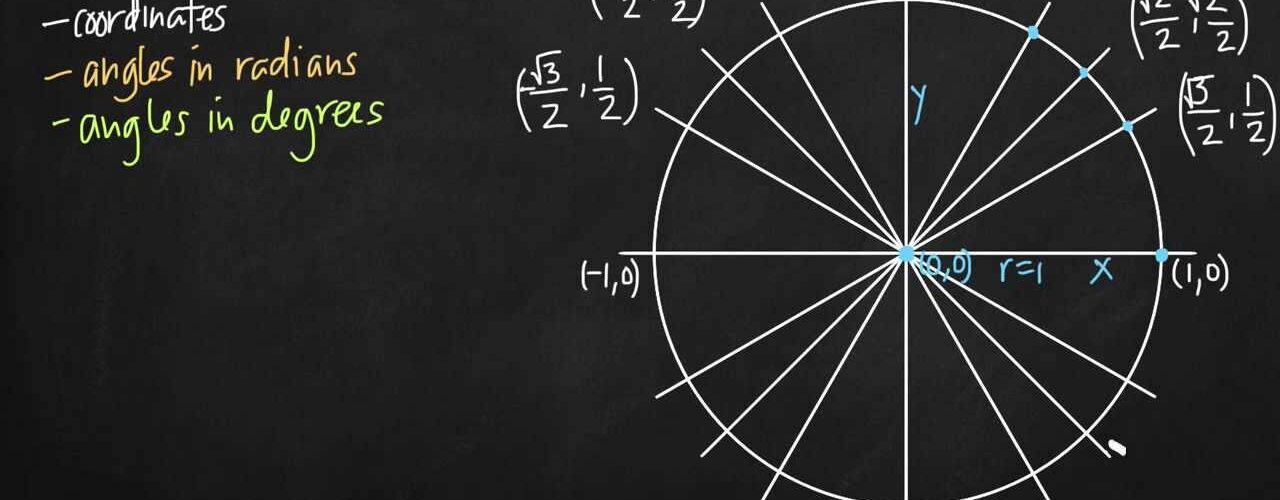


Add comment