Integrals are one of the two fundamental operations in calculus (the other being differentiation). While derivatives deal with rates of change, integrals provide a way to compute the accumulation of quantities, such as areas under curves. In this article, we will explore the core concepts behind integrals, how to calculate them, and their real-world applications.
What is an Integral?
An integral in calculus represents the accumulation of quantities, like areas, volumes, or total change. It is the reverse operation of differentiation, known as anti-differentiation. The process of finding an integral is called integration.
There are two main types of integrals:
- Definite Integrals: These compute the accumulation of a quantity over an interval and result in a specific number.
- Indefinite Integrals: These represent a family of functions and include a constant of integration.
Definite Integral
The definite integral of a function

This integral gives the net area under the curve of
Indefinite Integral
The indefinite integral of a function
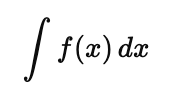
This represents a family of functions whose derivative is
Fundamental Theorem of Calculus
The Fundamental Theorem of Calculus links the concepts of differentiation and integration, providing a powerful framework for calculating definite integrals.
The theorem consists of two parts:
- First Fundamental Theorem of Calculus: If
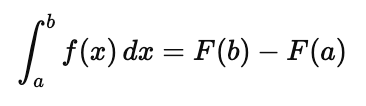
In other words, to calculate the definite integral, you can find an antiderivative of the function and evaluate it at the endpoints of the interval.
- Second Fundamental Theorem of Calculus: It states that if

This shows that differentiation and integration are inverse operations.
How to Calculate Integrals
Basic Rules of Integration
Integration follows several important rules that simplify the calculation of integrals. These are analogous to the rules of differentiation:
Constant Rule:
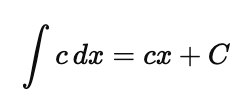
Where
Power Rule:
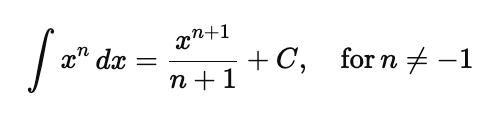
This is the reverse of the power rule in differentiation.
Sum Rule:
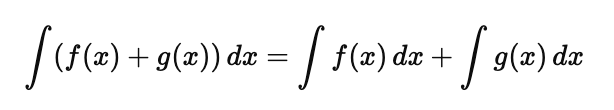
Constant Multiple Rule:

Substitution Rule (for more complex integrals):
If
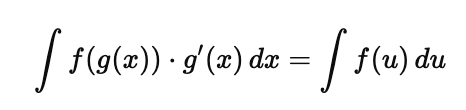
This is also known as u-substitution and is useful for integrals involving composite functions.
Example: Finding an Indefinite Integral
Let’s calculate the indefinite integral of
Using the power rule for each term:
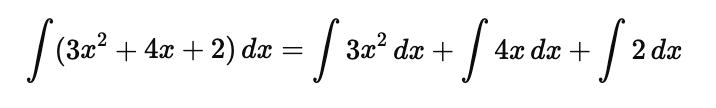
Integrating term by term:
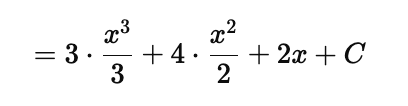
Simplify:
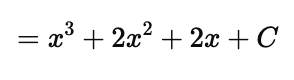
Thus, the indefinite integral is:
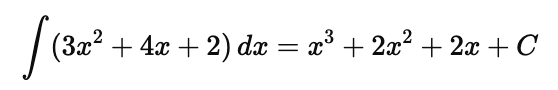
Example: Finding a Definite Integral
Now let’s calculate the definite integral of
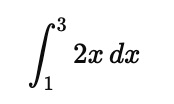
Step 1: Find the indefinite integral of
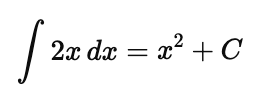
Step 2: Apply the Fundamental Theorem of Calculus.
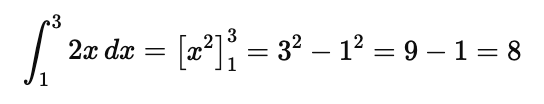
Thus, the area under the curve
Applications of Integrals
Integrals have a wide range of applications across various fields, from mathematics and physics to economics and engineering. Here are some of the most common applications:
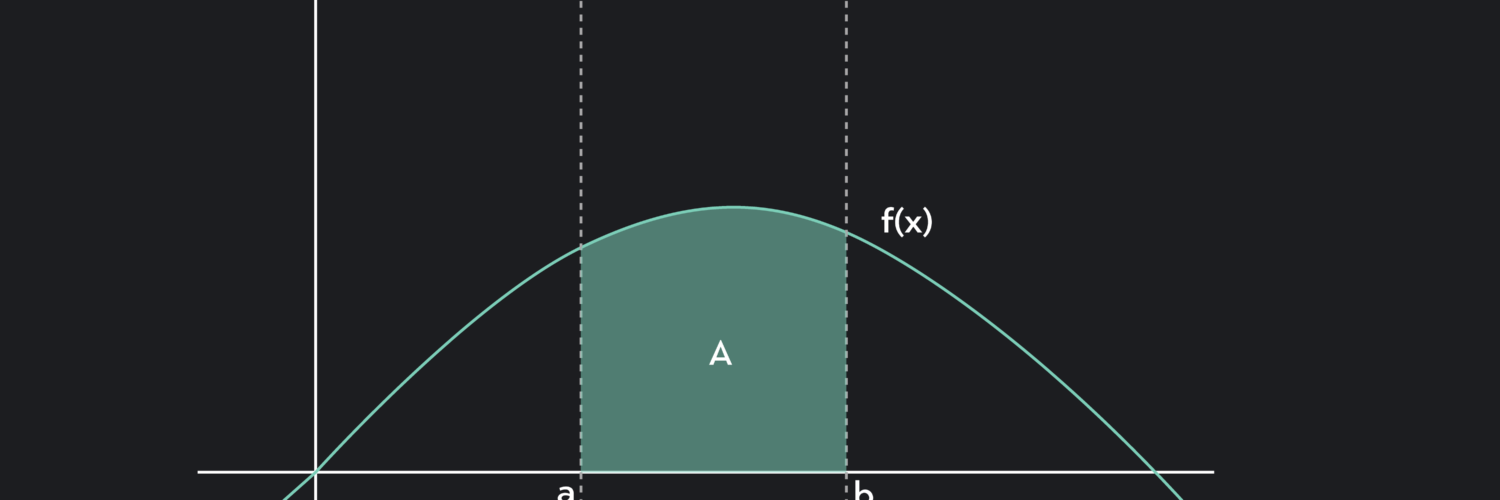
1. Area Under a Curve
One of the primary uses of definite integrals is finding the area under a curve. For a function

This is useful in a variety of real-world situations, such as calculating the total distance traveled by an object if you know its velocity over time.
2. Volume of Solids
Integrals are used to calculate the volume of solids, particularly when dealing with three-dimensional shapes like cylinders, cones, or spheres. A common method is using the disk method or shell method to compute the volume of a solid of revolution.
For example, the volume of a solid formed by revolving a function
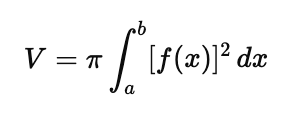
3. Physics: Work and Energy
In physics, integrals are used to compute work, which is the total force applied over a distance. If the force
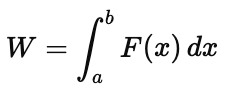
This is essential for understanding how energy is transferred in systems.
4. Economics: Consumer and Producer Surplus
In economics, integrals help determine consumer surplus and producer surplus, which represent the benefit consumers and producers derive from market transactions.
- The consumer surplus is the area between the demand curve and the price level.
- The producer surplus is the area between the supply curve and the price level.
Common Mistakes to Avoid
- Forgetting the Constant of Integration: When solving indefinite integrals, always include the constant of integration
- Incorrect Use of the Power Rule: Be careful when applying the power rule. For instance, the power rule does not apply when
- Not Simplifying the Integral Before Applying Rules: Some integrals can be simplified through algebraic manipulation or by using trigonometric identities. Always check if simplifications can make the integration process easier.
Conclusion
Integrals are a fundamental concept in calculus that allow us to calculate areas, volumes, and the accumulation of quantities. Whether you are solving problems in mathematics, physics, economics, or engineering, integrals provide powerful tools for analyzing continuous change. By mastering the rules of integration and understanding their applications, you can tackle a wide range of real-world problems and deepen your mathematical knowledge.

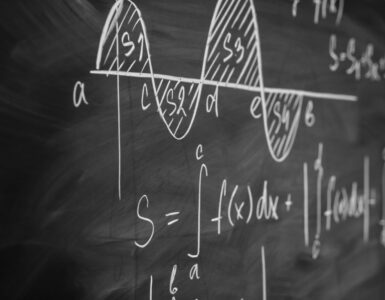
 Free Tutoring – By Students, For Students
Free Tutoring – By Students, For Students Get completely free, personalized tutoring from high school and college students who understand what it’s like to be a learner today.
Get completely free, personalized tutoring from high school and college students who understand what it’s like to be a learner today.

Add comment